Aug 10, 2023
Technology advance could expand the reach of 3D nanoprinting
Posted by Dan Breeden in categories: 3D printing, augmented reality, biological, computing, nanotechnology
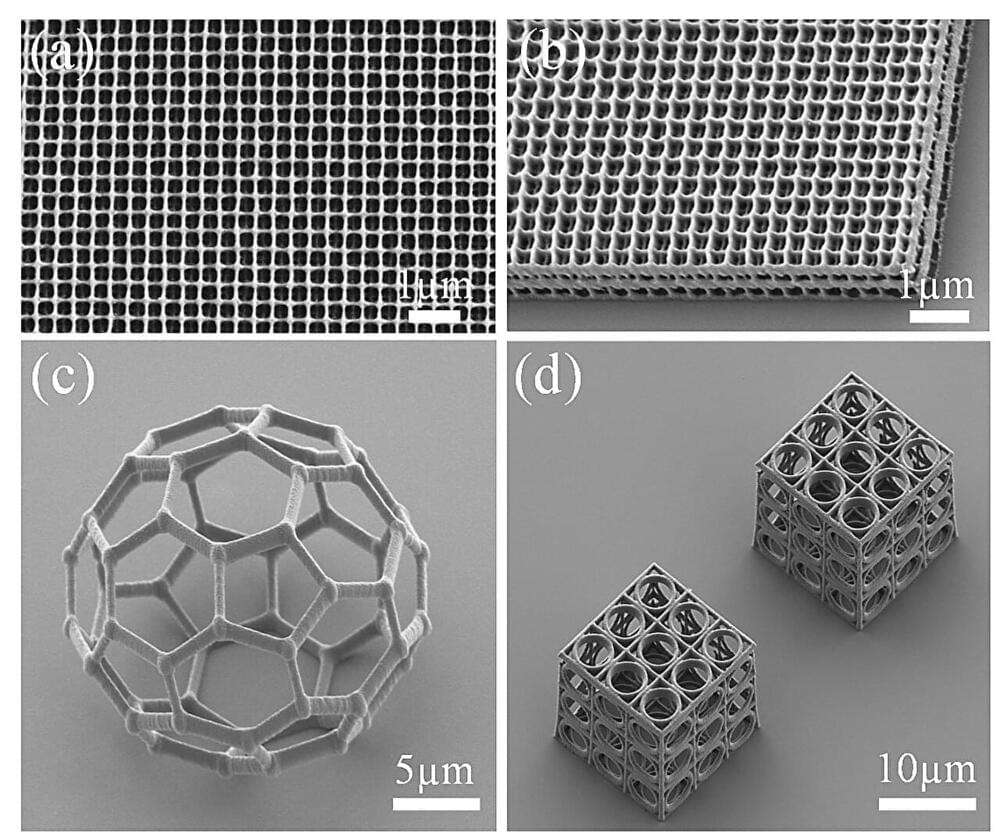
Researchers have developed an easy-to-build, low-cost 3D nanoprinting system that can create arbitrary 3D structures with extremely fine features. The new 3D nanoprinting technique is precise enough to print metamaterials as well as a variety of optical devices and components such as microlenses, micro-optical devices and metamaterials.
“Our system uses a two-step absorption process to realize 3D printing with accuracy reaching the nanometer level, which is suitable for commercial manufacturing,” said research team leader Cuifang Kuang from the Zhejiang Lab and Zhejiang University, both in China. “It can be used for a variety of applications such as printing micro or nanostructures for studying biological cells or fabricating the specialized optical waveguides used for virtual and augmented reality devices.”
Conventional high-resolution 3D nanoprinting approaches use pulsed femtosecond lasers that cost tens of thousands of dollars. In Optics Letters, Kuang and colleagues describe their new system based on an integrated fiber-coupled continuous-wave laser diode that is not only inexpensive but also easy to operate.