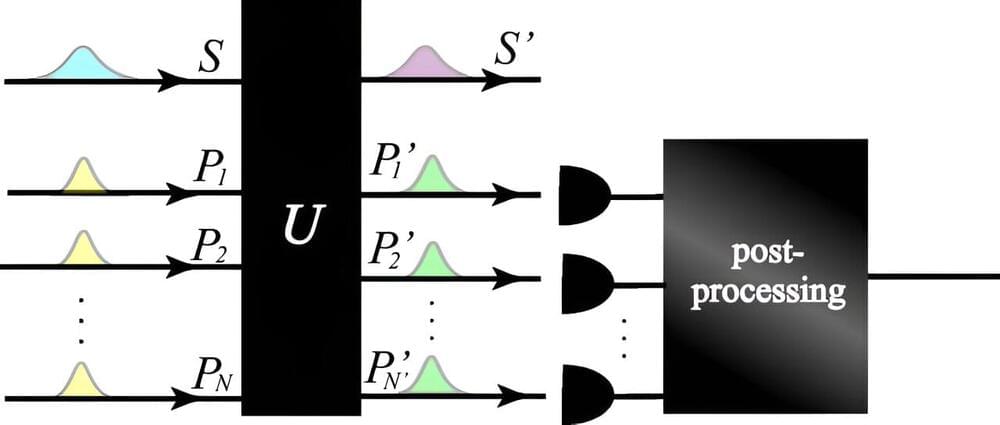
A study published in the journal Physical Review Letters by researchers in Japan solves a long-standing problem in quantum physics by redefining the uncertainty principle.
Werner Heisenberg’s uncertainty principle is a key and surprising feature of quantum mechanics, and he can thank his hay fever for it. Miserable in Berlin in the summer of 1925, the young German physicist vacationed on the remote, rocky island of Helgoland, in the North Sea off the northern German coast. His allergies improved, and he was able to continue his work trying to understand the intricacies of Bohr’s model of the atom, developing tables of internal atomic properties, such as energy, position and momentum.
When he returned to Göttingen, his advisor, Max Born, recognized these tables could each be formed into a matrix—essentially a two-dimensional table of values. Together with the 22-year-old Pasqual Jordan, they refined their work into matrix mechanics—the first successful theory of quantum mechanics—the physical laws that describe tiny objects like atoms and electrons.